Mindset Mathematics, and What's the Point of Math?

I’ve recently purchased two math books that I’m really happy with, so I’m sharing them with you: Mindset Mathematics and What's the Point of Math? I haven’t changed Wanda’s math curriculum—that’s still Beast Academy, and I still love it. These are some extra resources for fun & deepening her understanding.

Mindset Mathematics
This is a series of books, aligned to grade levels Kindergarten through grade 8, and designed to provide great learning for every kid, whether they've excelled at math or have struggled with it. The books are from Jo Boaler of Stanford, who is a big advocate for approaching math creatively, with a bunch of growth mindset learning woven in, to reach populations of kids who have been getting the “math isn’t for me” message from current math teaching methods. She has a lot of science to back her up, she works closely with neuroscientists and education researchers in her work at Stanford.
The book has a series of “Big Idea” activities, where kids are presented with math concepts in visual ways, and do related hands-on activities that stimulate stronger mental maps of how numbers work. That comes with a lot of exploration and discussion. Math tends to be a heads-down, do-the-worksheet kind of subject, and this breaks away from that, and lets it feel more like a fun science experiment, or maybe even arts & crafts. The activities are designed to be accessible no matter where a kid is at, mathematically speaking. Kids who are comfortable with math will have an opportunity to go deep and get into fun, chewy ideas about numbers, while kids who have been more math-averse will hopefully find the activity inviting and relatively comfortable, and will be able to unlock that same deep understanding in fresh ways.
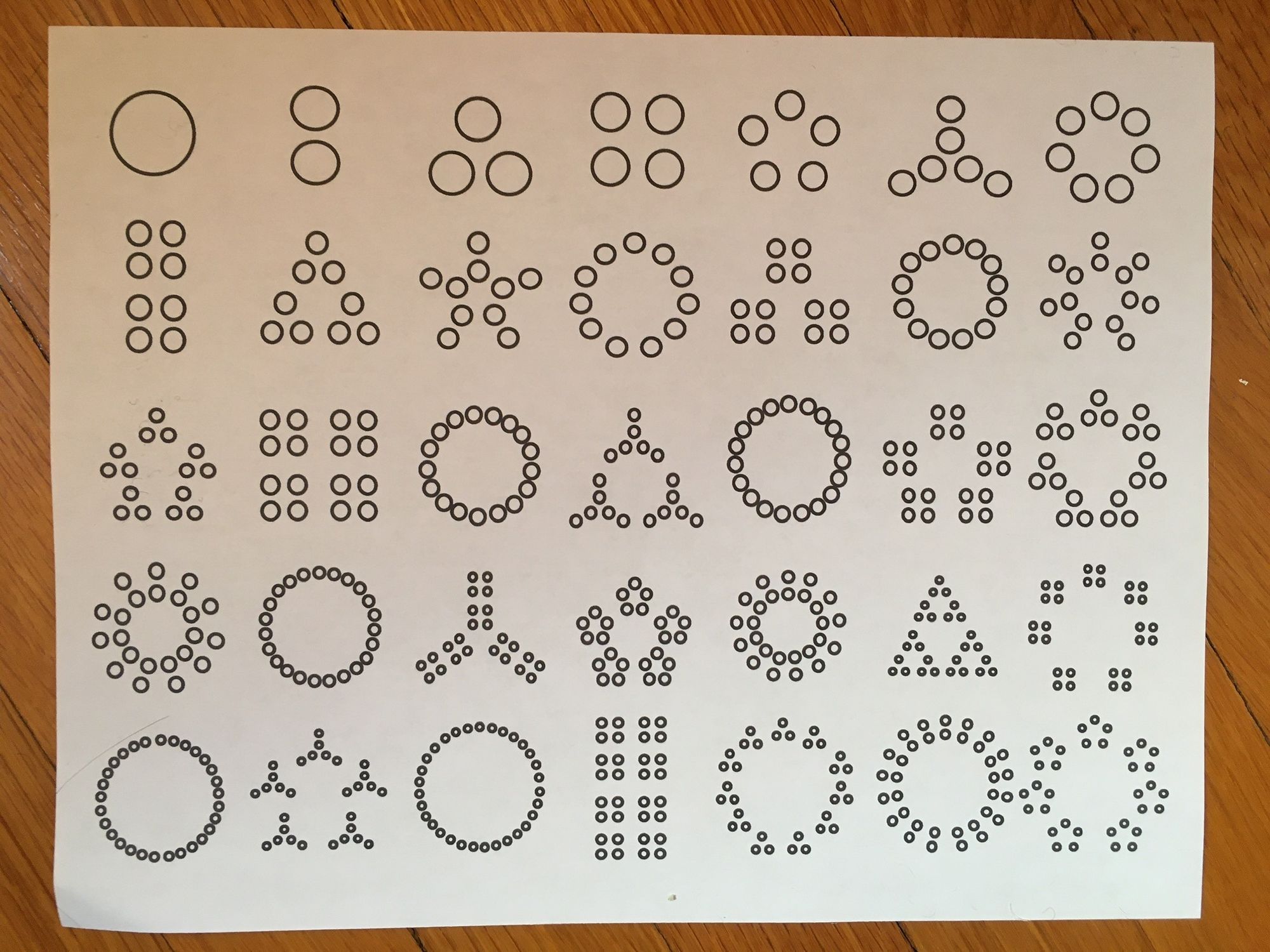
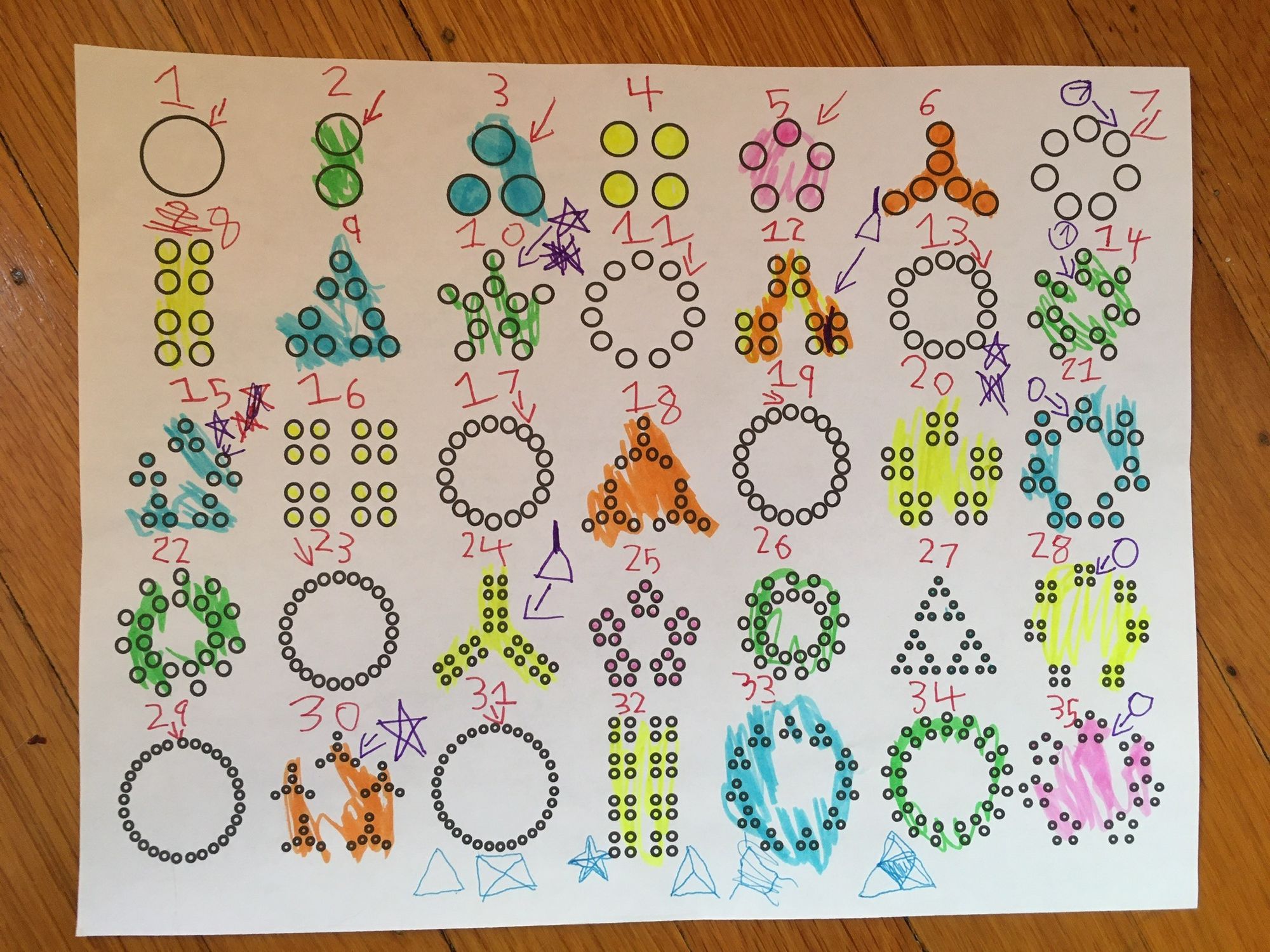
In today’s activity, Wanda was given a piece of paper that had a series of clusters of dots, and I asked her what she noticed about it. She identified that the clusters were representing the numbers 1-35, in sequence. I then asked her to spend some time seeing if she could identify patterns, and color coding them with highlighters, and we discussed together what she was noticing. I’ve attached what she came up with. She quickly identified that there were clusters within the clusters, and these represented factors of the number, and appeared in other numbers. She soon identified that there was also an overall shape to the clusters, which represented the other factor needed to get that number. She spotted a bunch of other patterns, and predicted what the shapes further out in the sequence might look like. For example, she was able to tell that the numbers represented by clusters of paired dots were not just multiples of two, but that the number being multiplied was always an odd number, but some were skipped… when she later identified the prime numbers on the sheet, a light bulb went off, and she was able to see that had been the pattern in those numbers.
Wanda is not at all new to multiplication; she’s up to exponents now in her main math curriculum. But she loved exploring the factors and multiples in this way, seeing patterns emerge—plus, it was fun for her to get to think more about prime numbers, since they get lost in the shadows when you’re doing multiplication. She loved this activity.
Another, earlier activity we did from this book was about honing your math discussion chops. It posits that there are three levels of convincing somebody: convincing yourself, convincing a friend, and convincing a skeptic. It’s easiest to convince yourself of something. It’s slightly harder to convince a friend, but they’re inclined to believe you because they like you. The hardest, and the goal, is to be able to convince a skeptic. In the activity, you make a geometric shape out of folded paper, and then support your argument that she shape is what you say it is, using what you know about shapes. The person you’re paired with plays the role of a skeptic, challenging: what makes this a square, and not a rhombus? how do you know that’s a 90 degree angle? how do you know that is an isosceles triangle? We took turns being the skeptic. By the end of the activity, Wanda was able to see the value in specifics, measurements, and how “because it looks like it” doesn’t cut it. (This turned out to be an EXCELLENT foundation for the science work we’re doing right now, which is learning all about Carl Sagan’s Baloney Detection Kit) It really moved the needle on Wanda understanding the value in explaining herself in specific terms.


What's the Point of Math?
It’s a fair question, and every kid asks it! I haven’t actually read through this book yet, but Wanda has been picking it up repeatedly, and when our Mindset Mathematics activity today turned to prime numbers, she excitedly leapt up to open to the prime numbers page in this book. It’s from DK, full color with easily-digestible graphics and text, chock full of real-world ways that math makes cool things possible. Dozens of examples, like how Ancient Egyptians measured the area of crop land, and how Florence Nightingale developed the pie chart to clearly show that many war deaths were due to unsanitary conditions and were preventable.